MAIC Micro Move #1
- Yay Math!
- Feb 5, 2019
- 2 min read
As many of you know we've been visiting classrooms for the past few weeks. We want to extend a heartfelt thank you to all the teachers who have opened their doors and all the students who have shared their thinking with us. We have learned so much from each of you! So much in fact, that we felt we needed to add a new type of post to the MAIC blog. The MAIC Micro Move – a spotlight on those small but mighty teacher moves that are actually the foundation to building an amazing classroom learning culture.
The first to receive this small but significant honor is Lauren and her 6th grade class. Here's the move.
Lauren's class was in the midst of making sense of a table they had started the day before in response to this problem: "Can you help Crystal figure out the reduced price of chewy fruit worms given this information?"
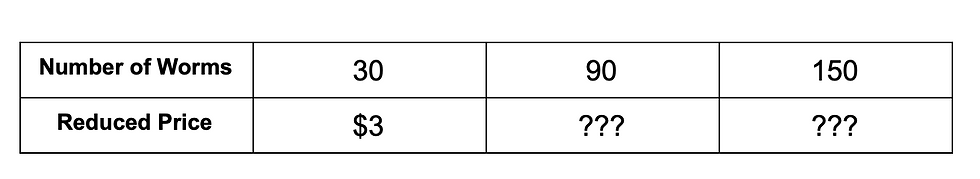

Lauren posed the question, "We came up with an interesting prediction didn't we? Javier thought that 150 would correspond to 15 in the table. Why did he think that? Talk to a neighbor.
The students turned to their partners and shared their thinking. When Lauren brought them back together a number of students wanted to share their thinking with the whole class. After two students shared some preliminary ideas, circling around the idea of a unit rate, one student went up and concisely described how he had determined the unit rate and how you could use it to predict the value for 15. I followed every word he said perfectly. His logic was beautiful. As I was busy being impressed by his reasoning, Lauren, causally leaning against a table at the side of the room said in a neutral tone, "Hmmm, I think I'm here," and she held her thumb up sideways, "what about the rest of you?"
As I scanned the student thumbs in the classroom, it was immediately apparent that what had felt crystal clear to me, was anything but to the students. Most had thumbs in the sideways position, many had their thumbs down, and only a few had their thumbs up.
What Lauren knew, but what was not immediately apparent to me, was that just because a student hears something explained beautifully– by a teacher or another student – it does not mean that they necessarily understand what was said. A perfect explanation of a mathematical concept by one student means that ONE student understands. Not a whole class full. She followed up her question with another pair share, asking those with sideways thumbs to share first. Then she asked if anyone had follow-up questions for the presenter.
The reason this move was so powerful was that she modeled skepticism and normalized confusion. She stated it clearly and visually (with her thumb) and implicitly gave every student in her class permission to admit their own partial understanding. It was a small but powerful teaching move.
Comments